The ACT will probably include some math problems that involve inverse proportionality. Inverse proportionality refers to a connection between two variables based on either multiplication or division, where the variables tend to rise and fall separately. That is, as one increases, the other decreases, and vice versa.
Two variables, x and y, are inversely proportional when the following equation is true for some constant k:
xy = k
Inverse proportionality means that as the value of one variable changes, the other value must also change so that any resulting product xy remains constant.
Example 1
Two variables p and q are inversely proportional, such that if p = 4, then q = 8. What is the value of q when p = 16?
(A) 1
(B) 2
(C) 4
(D) 16
(E) 32
The product pq is a constant and
Thus, pq = 32 for all possible pairings of p and q. Substitute 16 for p into this equation:
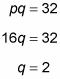
Therefore, the correct answer is Choice (B).
Example 2
If
and uv = 10, which of the following must be true?
(F) t and u are inversely proportional
(G) t and v are inversely proportional
(H) t and w are directly proportional
(J) t and w are inversely proportional
(K) u and v are directly proportional
Begin by cross-multiplying:
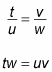
Substitute 10 for uv:
tw = 10
Thus, tw = k for k = 10, so t and w are inversely proportional. So the correct answer is Choice (J).





