You will need to know how to work with decimals for the ASBAB. Decimals are a method of writing fractional numbers without using a numerator and denominator. You can write the fraction 7/10 as the decimal 0.7; you pronounce it “seven-tenths” or “zero point seven.” The period or decimal point indicates that the number is a decimal.
Other decimals exist, such as hundredths or thousandths. They’re all based on the number ten, just like our number system:
0.7: Seven-tenths

0.07: Seven-hundredths

0.007: Seven-thousandths

0.0007: Seven-ten-thousandths

0.00007: Seven-hundred-thousandths

If a decimal is less than 1, it’s traditional in mathematics to place a zero before the decimal point. Write “0.7” not “.7”
A decimal may be greater than one. The decimal 3.7 would be pronounced as “three and seven-tenths”
Converting decimals to fractions
To convert a decimal to a fraction, write all the digits following the decimal point in the numerator. If you see zeros before any nonzero digits, you can ignore them.
The denominator is always a one followed by zeros. The number of zeros in the denominator is determined by the total number of digits to the right of the decimal point (including the leading zeros):
One digit: Denominator = 10. Example:

Two digits: Denominator = 100. Example:

Three digits: Denominator = 1,000. Example:

Four digits: Denominator = 10,000. Example:
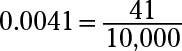
Of course, you can also convert fractions to decimals.
Adding and subtracting decimals
You add and subtract decimals just as you do regular numbers (integers), except that before you perform your operation, you arrange the numbers in a column with the decimals lined up one over the other.
Add the numbers 3.147, 148.392, and 0.074.
Put the numbers in an addition column with the decimals lined up and perform the addition:

Rounding
Rounding a number means limiting a number to a few (or no) decimal places. For example, if you have a $1.97 in change in your pocket, you may say, “I have about $2.” The rounding process simplifies mathematical operations.
To round a number, you first determine what place you’re rounding to. For example, the math subtests that make up the AFQT may ask you to round to the nearest tenth. Then look at the number immediately to the right of that place.
If the number is 5 or greater, round the digit to the left up; for any number under five, round the digit to the left down. Thus, you’d round 1.55 up to 1.6 and 1.34 down to 1.3.
You can also round other numbers, such as whole numbers. For example, 1,427 becomes 1,400 when you round to the nearest 100. However, most of the rounding operations you encounter on the Mathematics Knowledge subtest involve rounding decimals to the nearest tenth or nearest hundredth.
Percents
Percent literally means “part of 100.” That means, for example, that 25 percent is equal to 25/100, which is equal to 0.25.
If a problem asks you to find 25 percent of 250, it’s asking you to multiply 250 by 0.25.
To convert a percent to a decimal number, remove the percentage sign and move the decimal point two places to the left: 15 percent is 0.15, and 15.32 percent is 0.1532. Conversely, to change a decimal number to percent, add the percentage sign and move the decimal point two places to the right: 4.321 is equal to 432.1 percent.





