In physics, when you go from linear motion to rotational motion, you need to change the equations that you use to calculate your results. Here are the angular equivalents (or analogs) for the linear motion equations:
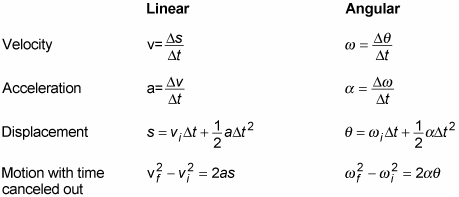
In all these equations, t stands for time,
f means final, and i means initial. In the linear equations, v is velocity, s is displacement, and a is acceleration. In the angular equations,
You know that the quantities displacement, velocity, and acceleration are all vectors; well, their angular equivalents are vectors, too. First, consider angular displacement,
this is a measure of the angle through which an object has rotated. The magnitude tells you the size of the angle of the rotation, and the direction is parallel to the axis of the rotation. Similarly, angular velocity,
has a magnitude equal to the angular speed and a direction that defines the axis of rotation. The angular acceleration,
has a magnitude equal to the rate at which the angular velocity is changing; it’s also directed along the axis of rotation.
If you consider only motion in a plane, then you have only one possible direction for the axis of rotation: perpendicular to the plane. In this case, these vector quantities have only one component — this vector component is just a number, and the sign of the number indicates all you need to know about the direction. For example, a positive angular displacement may be a clockwise rotation, and a negative angular displacement may be a counterclockwise rotation.
Just as the magnitude of the velocity is the speed, the magnitude of the angular velocity is the angular speed. Just as the magnitude of a displacement is a distance, the magnitude of an angular displacement is an angle — that is, the magnitude of the vector quantity is a scalar quantity.

