Calculating Heat Exchange in a Closed System

In physics, you can calculate how much heat energy is required to raise an object of a certain mass by a certain temperature — all you need is the object’s specific heat.

At a given temperature, different materials can hold different amounts of thermal energy. For instance, if you warm up a potato, it can hold its heat longer (as your tongue can testify) than a lighter material such as cotton candy. Why? Because the potato stores more thermal energy for a given change in temperature; therefore, more heat has to flow to cool the potato than is needed to cool the cotton candy. The measure of how much heat it takes to raise the temperature of an object of a given mass a given number of degrees is called its specific heat.

Suppose you see someone making a pot of coffee. You measure exactly 1.0 kilogram of brewed coffee in the pot, and then you get down to the real measurements. You find out that you need 4,186 joules of heat energy to raise the temperature of the coffee by 1 degree Celsius, but you need only 840 joules to raise 1.0 kilogram of glass by 1 degree Celsius; the coffee and glass have different specific heats. The heat energy goes into the substance being heated, which stores the energy as internal energy until it leaks out again. (Note: If you need 4,186 joules to raise 1.0 kilogram of coffee by 1 degree Celsius, you need double that, 8,372 joules, to raise 2.0 kilograms of coffee by 1 degree Celsius or to raise 1.0 kilogram of coffee by 2 degrees Celsius.)

The following equation relates the amount of heat needed to raise an object’s temperature to the change in temperature and the amount of mass involved:

Here, Q is the amount of heat energy transferred into the object (measured in joules if you’re using the MKS, or meter-kilogram-second, system), m is the mass of the object,

(measured in degrees Celsius or kelvin), and c is a constant called the specific heat, which is measured in joules per kilogram-degree Celsius,

Usually physicists calculate the specific heat through experimentation, so most problems give you c or refer you to a table of specific-heat values for various materials.

You can use the heat equation to find out how temperature changes when you mix liquids of different temperatures. Suppose you have 45 grams of coffee in your cup, but it cooled while you were figuring out the coffee’s specific heat. You call over your host. The coffee is 45 degrees Celsius, but you like it at 65 degrees Celsius. The host gets up to pour some more. “Just a minute,” you say. “The coffee in the pot is 95 degrees Celsius. Wait until I calculate exactly how much you need to pour.”

The following equation represents the heat lost by the new mass of coffee, m₁:

And here’s the heat gained by the existing coffee, mass m₂:

Assuming you have a superinsulating coffee mug, no energy leaves the system to the outside, and because energy cannot be created or destroyed, energy is conserved within such a closed system; therefore, the heat lost by the new coffee is the heat that the existing coffee gains, so

Therefore, you can say the following:

cm₁(T – T_1,0) = –cm₂(T – T_2,0)

Dividing both sides by the specific heat of coffee, c, and plugging in the numbers gives you the following:

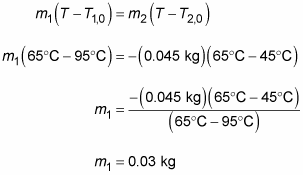
You need 0.03 kilograms, or 30 grams. Satisfied, you put away your calculator and say, “Give me exactly 30 grams of that coffee.”