The isosceles right triangle, or the 45-45-90 right triangle, is a special right triangle. The two acute angles are equal, making the two legs opposite them equal, too. What’s more, the lengths of those two legs have a special relationship with the hypotenuse (in addition to the one in the Pythagorean theorem, of course).
In an isosceles right triangle, if the legs are each a units in length, then the hypotenuse is
or about 1.4 times as long as a leg.
Now that you know how isosceles right triangles work, try your hand at this sample problem: If an isosceles right triangle has a hypotenuse that’s 16 units long, then how long are the legs?
Create an equation to solve.
The hypotenuse is

where a is the length of the legs. You know that the hypotenuse is 16, so you can solve the equation
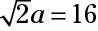
for the length of a.
Solve for a.
Divide each side by the radical to get
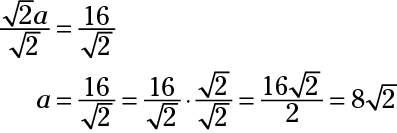
Each leg is about 11.3 units.

