Mathematical problems that require the use of trig functions often have one of two related angles: the angle of elevation or the angle of depression. The scenarios that use these angles usually involve calculating distances that can't be physically measured.
For example, these angles are used when finding the distance from an airplane to a point on the ground or the distance up to a balloon or another object above you. Use the trig functions to solve for the missing part of the ratio or the side of the imaginary right triangle.
An angle of elevation is measured from the horizontal going upward. The horizontal is usually the ground, street, floor, or any other flat object. Even though the ground isn't perfectly flat or horizontal, you determine the measurements with the assumption that it is. In trig, you have to consider the optimal situation — focus on the big picture, rather than on the imperfections.
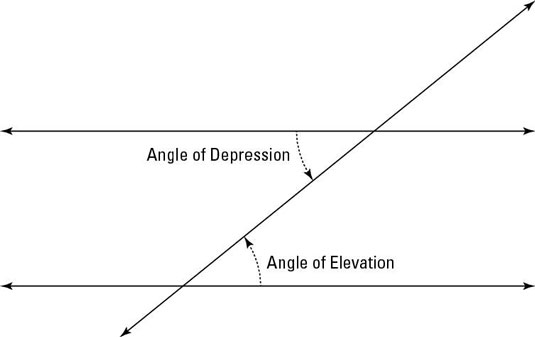
An angle of depression is measured from the horizontal going downward. For these angles, the horizontal is, for example, an airplane's flight path or a person's line of sight while standing on a mountaintop. The angle is formed when a person on that plane or mountaintop looks at an object on the ground (or on a path parallel to the ground).




