In quantum physics, you’ll often work with Hermitian adjoints. The Hermitian adjoint — also called the adjoint or Hermitian conjugate — of an operator A is denoted
To find the Hermitian adjoint, you follow these steps:
Replace complex constants with their complex conjugates.
The Hermitian adjoint of a complex number is the complex conjugate of that number:

Replace kets with their corresponding bras, and replace bras with their corresponding kets.
You have to exchange the bras and kets when finding the Hermitian adjoint of an operator, so finding the Hermitian adjoint of an operator is not just the same as mathematically finding its complex conjugate.
Replace operators with their Hermitian adjoints.
In quantum mechanics, operators that are equal to their Hermitian adjoints are called Hermitian operators. In other words, an operator is Hermitian if

Hermitian operators have special properties. For instance, the matrix that represents them can be diagonalized — that is, written so that the only nonzero elements appear along the matrix’s diagonal. Also, the expectation value of a Hermitian operator is guaranteed to be a real number, not complex.
Write your final equation.

Here are some relationships concerning Hermitian adjoints:
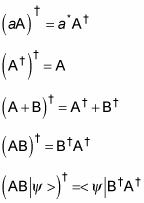
Remember that the asterisk symbol (*) means the complex conjugate. A complex conjugate flips the sign connecting the real and imaginary parts of a complex number.





