In physics, you can use Boyle’s and Charles’s laws to express the ideal gas law in different ways. For example, you can express the relationship between the pressure and volume of an ideal gas before and after one of those quantities changes at a constant temperature like this:
PfVf = PiVi
This equation, called Boyle’s law, says that all other factors being the same, the product of pressure and volume (PV) will be conserved.
At constant pressure, you can say that the following relationship is true for an ideal gas:
This equation, called Charles’s law, says that the ratio of volume to temperature (V/T) will be conserved for an ideal gas, all other factors being the same.
Here’s an example that uses Boyle’s law. You’re taking a well-deserved vacation on the beach when the director of the Acme Tourism Company runs up to you and says, “We usually let our tourists scuba dive at 10.0 meters for ten minutes, but one of our tourists says he wants to stay down for a half an hour. He’s going to run out of air, and we’ll be sued!”
“Hmm,” you say. “Let me have your data.” You take some papers from the director and see that the Acme Tourism Company has scuba tanks with a volume of 0.015 cubic meters that are pressurized to
The diver breathes at a rate of 0.04 cubic meters per minute.
Because you’re a physicist, you happen to know how scuba tanks work. They keep the air in the lungs at the same pressure as the surrounding water pressure (or else the lungs may collapse).
The way to tackle this problem is to take the volume of pressurized air inside the tank and work out what its volume would be if it were all released at the pressure you find at the submerged depth. You know the oxygen requirements of the body as a volume rate, so you can work out how long the pressurized air can sustain breathing from the volume the air would have at the submerged pressure. To work out the volume the pressurized air in the tank would have when released at the submerged depth, you use Boyle’s law:
PfVf = PiVi
You know the pressure of the scuba tank and its volume. Now you need to know the pressure at the diver’s depth to be able to calculate the available volume of air for the diver. You can find the water pressure with this equation,
where Pw is the pressure of the water,
g is the acceleration due to gravity, and h is the change in depth:
The density of water is about 1,025 kilograms per cubic meter, and the diver descends 10.0 meters, so you have the following pressure from the water:
To get the total pressure at the diver’s depth, add the pressure of air on the surface of the water — that is, atmospheric pressure, Pa — to the water pressure:
Now you’re ready to use Boyle’s equation:
PfVf = PiVi
where Pi is the pressure of the tank and Vi is the volume of the tank.
You want to find the volume of air available to the diver, so solve for Vf:
Plugging in the numbers and doing the math gives you the volume of air available:
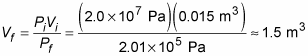
How long will that last? The diver breathes at a rate of 0.04 cubic meters per minute, so 1.5 cubic meters of air is enough for
You tell the director that the diver will have enough air for 38 minutes. The director breathes a sigh of relief. “No lawsuit, then?”
“No lawsuit.”
“But what if the diver goes down to 30.0 meters?” You figure out the new pressure from the water at 30 meters:
And add air pressure to get
As before, you want to find Vf, the volume of air available to the diver:
Plugging in the numbers and doing the math gives you this result:
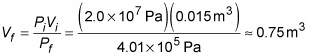
At a rate of 0.04 cubic meters per minute, 0.75 cubic meters of air is enough for
“Uh oh,” you tell the director.
“Lawsuit?” the director asks.
“Lawsuit.”





