Using principles of physics, if you push a partially open door in the same direction as you push a closed door, you create a different torque because of the non-right angle between your force and the door.
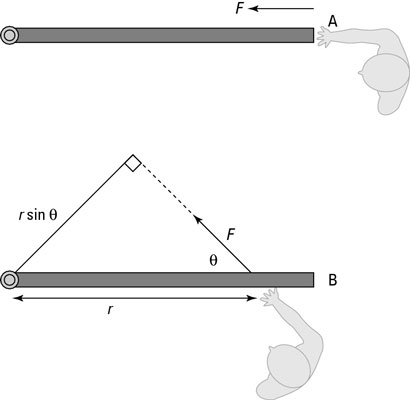
Take a look at diagram A in the figure to see a person obstinately trying to open a door by pushing along the door toward the hinge. You know this method won’t produce any turning motion, because the person’s force has no lever arm to produce the needed turning force. In this case, the lever arm is zero, so it’s clear that even if you apply a force at a given distance away from a pivot point, you don’t always produce a torque. The direction you apply the force also counts.
Generating torque is how you open doors, whether you have to quickly pop a car door or slowly pry open a bank-vault door. But how do you find out how much torque you generate? First, you calculate the lever arm, and then you multiply that lever arm by the force to get the torque.
Take a look at diagram B in figure. You apply a force to the door at some angle,
The force may open the door, but it isn’t a sure thing, because as you can tell from the figure, you apply less of a turning force here. What you need to do is find the lever arm first. As you can see in diagram B, you apply the force at a distance r from the hinge. If you apply that force perpendicularly to the door, the lever arm’s length would be r, and you’d get the torque (represented by the Greek letter tau):
However, that’s not the case here, because the force isn’t perpendicular to the door.
The lever arm is the effective distance from the pivot point at which the force acts perpendicularly. Picture moving the point where the force is applied, carrying the force vector along without changing its direction. When you move to a spot where the force is perpendicular to a line from the axis of rotation, the distance from that spot to the axis is the lever arm.
To see how this works, take a look at diagram B in the figure, where you can draw a lever arm from the pivot point so that the force is perpendicular to the lever arm. To do this, extend the force vector until you can draw a line from the pivot point that’s perpendicular to the force vector. You create a new triangle. The lever arm and the force are at right angles with respect to each other, so you create a right triangle. The angle between the force and the door is
and the distance from the hinge at which you apply the force is r (the hypotenuse of the right triangle), so the lever arm becomes
When
goes to zero, so does the lever arm, so there’s no torque (see diagram A in the figure). You know that
This is a general equation; if you apply a force with a magnitude of F at a distance r from a pivot point, where the angle between vector r and the force vector F is
the torque you produce will have a magnitude of
So, for example, if
F = 200 newtons, and r = 1.0 meter, you get
This number is less than you’d expect if you just push perpendicularly to the door (which would be 200 newton-meters).





