You can use the Stefan-Boltzmann constant to measure the amount of heat that is emitted by a blackbody. Physicists have determined that a blackbody is an object that absorbs 100 percent of the radiant energy striking it, and if it’s in equilibrium with its surroundings, it emits all the radiant energy as well.
In terms of reflection and absorption of radiation, most objects fall somewhere between mirrors, which reflect almost all light, and blackbodies, which absorb all light. The middle-of-the-road objects absorb some of the light striking them and emit it back into their surroundings. Shiny objects are shiny because they reflect most of the light, which means they don’t have to emit as much heat radiantly into the room as other objects. Dark objects appear dark because they don’t reflect much light, which means they have to emit more as radiant heat (usually lower down in the spectrum, where the radiation is infrared and can’t be seen).
So how much heat does a blackbody emit when it’s at a certain temperature? The amount of heat radiated is proportional to the time you allow — twice as long, twice as much heat radiated, for example. So you can write the heat relation, where t is time, as follows:
And as you may expect, the amount of heat radiated is proportional to the total area doing the radiating. So you can also write the relation as follows, where A is the area doing the radiating:
Temperature, T, has to be in the equation somewhere — the hotter an object, the more heat radiated. Experimentally, physicists found that the amount of heat radiated is proportional to T to the fourth power, T4. So now you have the following relation:
To show the exact relationship between heat and the other variables, you need to include a constant, which physicists measured experimentally. To find the heat emitted by a blackbody, you use the Stefan-Boltzmann constant,
which goes in the equation like this:
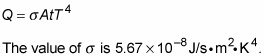
Note, however, that this constant works only for blackbodies that are perfect emitters.
Most objects aren’t perfect emitters, so you have to multiply by another constant most of the time — one that depends on the substance you’re working with. The constant is called emissivity, e, which is a pure number between 0 (for a perfect reflector) and 1 (for a perfect absorber).
The Stefan-Boltzmann law of radiation says the following:

the temperature in kelvins.
A person’s emissivity is about 0.98. At a body temperature of 37 degrees Celsius, how much heat does a person radiate each second? First, you have to factor in how much area does the radiating. If you know that the surface area of the human body is A = 1.7 m2, you can find the total heat radiated by a person by plugging the numbers into the Stefan-Boltzmann law of radiation equation, making sure you convert the temperature to kelvins:
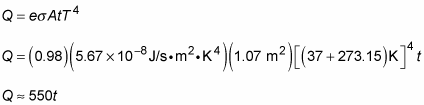
Then dividing both sides by t, you get
You get a value of 550 joules per second, or 550 watts. That may seem high, because skin temperature isn’t the same as internal body temperature, but it’s in the ballpark.





