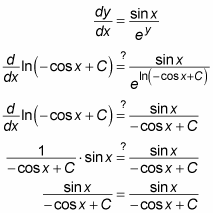Differential equations become harder to solve the more entangled they become. In certain cases, however, an equation that looks all tangled up is actually easy to tease apart. Equations of this kind are called separable equations (or autonomous equations), and they fit into the following form:
Separable equations are relatively easy to solve. For example, suppose that you want to solve the following problem:
You can think of the symbol
as a fraction and isolate the x and y terms of this equation on opposite sides of the equal sign:
eydy = sin x dx
Now integrate both sides:
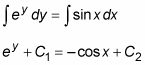
In an important sense, the previous step is questionable because the variable of integration is different on each side of the equal sign. You may think “No problem, it’s all integration!” But imagine if you tried to divide one side of an equation by 2 and the other by 3, and then laughed it off with “It’s all division!” Clearly, you’d have a problem. The good news, however, is that integrating both sides by different variables actually produces the correct answer.
C1 and C2 are both constants, so you can use the equation C = C2 – C1 to simplify the equation:
ey = –cos x + C
Next, use a natural log to undo the exponent, and then simplify:
ln ey = ln (–cos x + C)
y = ln (–cos x + C)
To check this solution, substitute this value for y into both sides of the original equation: