The HLR (Hypotenuse-Leg-Right angle) theorem — often called the HL theorem — states that if the hypotenuse and a leg of one right triangle are congruent to the hypotenuse and a leg of another right triangle, then the triangles are congruent. The following figure shows you an example.
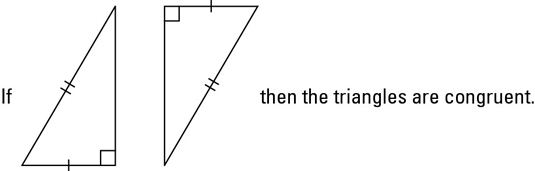
You can call this theorem HLR (instead of HL) because its three letters emphasize that before you can use it in a proof, you need to have three things in the statement column (congruent hypotenuses, congruent legs, and right angles).
Note: When you use HLR, listing the pair of right angles in a proof statement is sufficient for that part of the theorem; you don’t need to state that the two right angles are congruent.
Ready for an HLR proof? Well, ready or not, here you go.

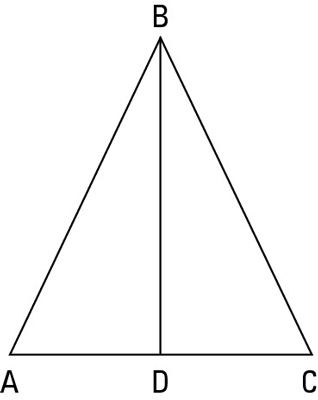
Here’s a possible game plan. You see the pair of congruent triangles and then ask yourself how you can prove them congruent. You know you have a pair of congruent sides because the triangle is isosceles.

Here’s the proof:
Statement 1:
Reason for statement 1: Given.
Statement 2:
Reason for statement 2: Definition of isosceles triangle.
Statement 3:
Reason for statement 3: Reflexive Property.
Statement 4:
Reason for statement 4: Given.
Statement 5:
Reason for statement 5: Definition of altitude.
Statement 6:
Reason for statement 6: Definition of perpendicular.
Statement 7:
Reason for statement 7: HLR (using lines 2, 3, and 6)
Statement 8:
Reason for statement 8: CPCTC.
Statement 9:
Reason for statement 9: Definition of midpoint.
Statement 10:
Reason for statement 10: Definition of median.

