The third trig function, tangent, is abbreviated tan. This function uses just the measures of the two legs and doesn’t use the hypotenuse at all. The tangent is described with this ratio: opposite/adjacent. No restriction or rule on the respective sizes of these sides exists — the opposite side can be larger, or the adjacent side can be larger.
So, the tangent ratio produces numbers that are very large, very small, and everything in between.
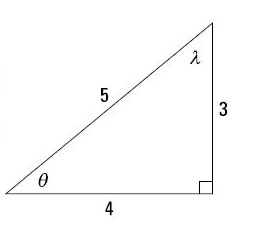
You see that the tangents are
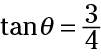
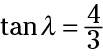
And in case you’re wondering whether the two tangents of the acute angles are always reciprocals (flips) of one another, the answer is yes.
The following example shows you how to find the values of the tangent for each of the acute angles in a right triangle where the hypotenuse is 25 inches and one leg is 7 inches.
Find the measure of the missing leg.
Using the Pythagorean theorem, a2 + b2 = c2, putting in 7 for a and 25 for c, and solving for the missing value, b, you find that the unknown length is 24 inches:

Select names for the acute angles in order to determine the opposite and adjacent designations.
The easiest way to do this is to draw a picture and label it.

The two acute angles are named with the Greek letters theta and lambda. The side opposite theta measures 7 inches, and the side adjacent to it measures 24 inches. For angle lambda, the opposite side measures 24 inches, and the adjacent side measures 7 inches.
Form the two tangent ratios by using the values 7, 24, and 25.






