Some equations on the ACT Math test may include one or more extra variables. Generally speaking, when an equation has more than one variable, you can’t solve it. As a result, in most cases, you must solve the equation in terms of the other variables — that is, isolate one variable on one side of the equation.
Example 1
In the equation 2pq + 5qr = 3pr, what is the value of p in terms of q and r?
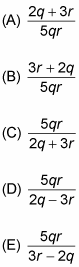
To answer this question, isolate p on one side of the equation. Begin by moving all the p terms to one side:
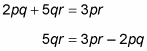
Now factor out p on the right side of the equation:
Divide both sides by 3r – 2q:
So the correct answer is Choice (E).
Sometimes when an equation has more than one variable, one variable drops out of the equation (surprise!), allowing you to solve for the remaining variable. Check out the following example to see how.
Example 2
What is the value of x in the equation 3(x + 4y) = 6(2y + 5)?
(F) 10
(G) 14
(H) 17
(J) 23
(K) Cannot be determined from the information given
At first glance, the equation doesn’t look solvable. But don’t be too quick to jump to this conclusion. You can, in fact, solve this problem. Begin by distributing to remove the parentheses:
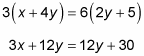
Now subtract 12y from both sides of the equation:
3x = 30
Magically, the y term has dropped out, leaving you with an equation that you can easily solve:
x = 10
The correct answer is Choice (F).
Finally, you can sometimes solve an equation with extra variables for an expression that includes both variables.
Example 3
If
what is the value of x – y?
(A) –2
(B) –1
(C) 1
(D) 2
(E) 4
This equation can’t be solved for either x or y. However, you can isolate the expression x – y on one side of the equation to answer the question. Begin by multiplying both sides by y – 1:
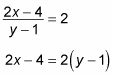
Now distribute on the right side of the equation:
2x – 4 = 2y – 2
Isolate the x and y terms on one side of the equation:
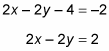
Factor out a 2 from each term on the left side:
Divide both sides by 2:
So the correct answer is Choice (C).






