In quantum physics, probabilities take the place of absolute measurements. Say you've been experimenting with rolling a pair of dice and are trying to figure the relative probability that the dice will show various values. You come up with a list indicating the relative probability of rolling a 2, 3, 4, and so on, all the way up to 12:
| Sum of the Dice | Relative Probability (Number of Ways of Rolling a Particular Total) |
|---|---|
| 2 | 1 |
| 3 | 2 |
| 4 | 3 |
| 5 | 4 |
| 6 | 5 |
| 7 | 6 |
| 8 | 5 |
| 9 | 4 |
| 10 | 3 |
| 11 | 2 |
| 12 | 1 |
In other words, you're twice as likely to roll a 3 than a 2, you're four times as likely to roll a 5 than a 2, and so on. You can assemble these relative probabilities into a vector (if you're thinking of a "vector" from physics, think in terms of a column of the vector's components, not a magnitude and direction) to keep track of them easily:
Okay, now you're getting closer to the way quantum physics works. You have a vector of the probabilities that the dice will occupy various states. However, quantum physics doesn't deal directly with probabilities but rather with probability amplitudes, which are the square roots of the probabilities. To find the actual probability that a particle will be in a certain state, you add wave functions of this state — which are going to be represented by these vectors — and then square them. So take the square root of all these entries to get the probability amplitudes:
That's better, but adding the squares of all these should add up to a total probability of 1; as it is now, the sum of the squares of these numbers is 36, so divide each entry by 361/2, or 6:
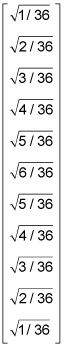
So now you can get the probability amplitude of rolling any combination from 2 to 12 by reading down the vector — the probability amplitude of rolling a 2 is 1/6, of rolling a 3 is
and so on.






